Summary
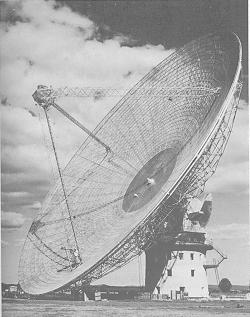 We have seen that geometry provides a means for focusing
co-axial electromagnetic radiation of all frequencies, in phase,
to a point. The paraboloid, which is defined as the set of points
equidistant from a fixed point (the focus) and
a plane, (the directrix) is the model for a passive (therefore
cheap) solution to the problems associated with communicating
over great distances with micro-waves.
We have seen that the problem of placing the feed head in the
path of the signal can be avoided by selecting, for the model,
an off-center section of the paraboloid. This geometric solution
has additional benefits, as we have noted. We have also shown
the effects of using a shallow or deep dish, and that antenna
gain is not dependent on the position of the focal point but only
on dish diameter (aperture) and wavelength of the radiation.
The backfire design was discussed and we saw that secondary reflectors
are typically either hyperbolic or elliptical in shape.
These geometric design solutions to electromagnetic wave problems
are satisfying for a number of reasons. The simple forms are pleasing
to the eye. The parabola has been defined and described in mathematics
texts for centuries. It has had applications in classical mechanics.
Electromagnetic wave theory is something quite new, yet parabolic
geometry is able to resolve difficulties here too.
We have seen that geometry provides a means for focusing
co-axial electromagnetic radiation of all frequencies, in phase,
to a point. The paraboloid, which is defined as the set of points
equidistant from a fixed point (the focus) and
a plane, (the directrix) is the model for a passive (therefore
cheap) solution to the problems associated with communicating
over great distances with micro-waves.
We have seen that the problem of placing the feed head in the
path of the signal can be avoided by selecting, for the model,
an off-center section of the paraboloid. This geometric solution
has additional benefits, as we have noted. We have also shown
the effects of using a shallow or deep dish, and that antenna
gain is not dependent on the position of the focal point but only
on dish diameter (aperture) and wavelength of the radiation.
The backfire design was discussed and we saw that secondary reflectors
are typically either hyperbolic or elliptical in shape.
These geometric design solutions to electromagnetic wave problems
are satisfying for a number of reasons. The simple forms are pleasing
to the eye. The parabola has been defined and described in mathematics
texts for centuries. It has had applications in classical mechanics.
Electromagnetic wave theory is something quite new, yet parabolic
geometry is able to resolve difficulties here too.
References

